Loi de Groupe pour les Unlabeled Necklaces?

[invite5a01804d]

Dear,

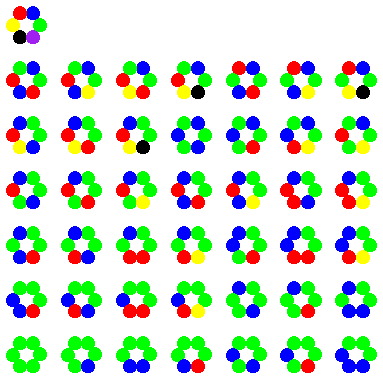
Let's consider the finite set of the unlabeled necklaces with 8 beads and possibly up to 8 colors UN(8,8).
They are known as unlabeled necklaces, invariant by permutation of the beads'color and by rotation.
There are 544 different such necklaces, named Ni, 0<i<543. Below the necklaces Ni are sorted by their minimum equivalent values in (0,8^8) :

N0 : (00000000)=(11111111)=(...)=(7 7777777) : the only necklace with 1 color
N1 : (00000001)=(10000000) rotation=(66666664) color permutation
N2 : (00000011)
N3 : (00000012)
N4 : (00000101)
...
...
N543 : (01234567) = the only necklace with 8 different colors

Question : Can a group structure by associated to this set?

I did my very best to sort the necklaces on a torus so that the torus look the most regular as possible, with the idea to use the group defined by the square-root-n of unity on the complex plane, but I failed.
For illustration, I have attached an image of UN(6,6).

The bibliography is quite difficult* : The p-adic representation and the Witt vector theory seem to be good candidate for doing so...
(maybe 7-adic here, because the first pearl of each collar is known (0) and because all the necklace but the latest have a maximum of 7 colors).

N. Metropolis and C.-C. Rota, Witt vectors and the Algebra of Necklaces, Adv. in Math. 50
(1983), 95–125
Cristian Lenart, Formal Group-Theoretic Generalizations of the Necklace Algebra, Including a q-Deformation, Journal of Algebra DOI:10.1006/jabr.1997.7203

Any experience or suggestion for further reading?

Thank
-----

[invite179e6258]

Hi,

since any set can be endowed with a group structure, I think you need to specify what properties you want the group operation to be compatible with.

[invite5a01804d]

Dear,

Thank you very much for this answer.
I am looking to sort the unlabeled necklaces in the most regular possible on a torus, i.e. so that only one (eventually 2) bead change between two consecutive necklaces.
Below is the graph matrix A of the unlabeled necklaces. Aij=1 if unlabeled necklaces i and j only differ by one bead. 0<=(i,j)<544.

There are n=Tr(A^544) loops that may yield to define n torus.

I am looking for the most "regular" torus. Any idea would be welcome!